Alternative Warp Speed Scale
At the risk–hopefully not too great–of offending Star Trek enthusiasts and traditionalists, it seems that a simple update to the Warp speed definitions would allow for more realistic exploration of space without departing unduly from the original concept.
The update would use a simple base-10 log scale. Log scales are often used in science and technology with Decibel and Richter scales being common examples.
Under this revision, a Starship’s speed or velocity (v) would be defined as:
| v = 10w-1c | …(1) |
| where: | |
| v = velocity (speed) | |
| w = Warp speed | |
| c = speed of light |
Equation 1 above uses w-1 (rather than just w) as the exponent to preserve Warp 1 as the speed of light (c), since 100 = 1, which is in keeping with existing scales and the general understanding of Warp speeds. Warp 2 becomes 10 times the speed of light, Warp 3 is 100 times, etc.
Table 1 is a comparison with existing Warp speed factors (multiples of the speed of light):
| Warp | TOS (w3) | TNG/DS9/VOY | Proposed (10w-1) |
|---|---|---|---|
| 1 | 1 | 1 | 1 |
| 2 | 8 | 10 | 10 |
| 3 | 27 | 39 | 100 |
| 4 | 64 | 102 | 1000 |
| 5 | 125 | 214 | 10,000 |
| 6 | 216 | 392 | 100,000 |
| 7 | 343 | 656 | 1M |
| 8 | 512 | 1024 | 10M |
| 9 | 729 | 1516 | 100M |
| 9.9 | – | 3053 | 794M |
| 9.99 | – | 7912 | 977M |
| 10 | Not possible | Not possible | 1000M (see below) |
Table 1 Comparison of Traditional and Proposed Warp Speed Factors
Series abbreviations:
- TOS = The Original Series
- TNG = The Next Generation
- DS9 = Deep Space Nine
- VOY = Voyager
The main advantages of the proposed approach are that it:
- aligns reasonably well, and in some cases better, with time/distance in the TV/film series
- makes the galaxy more navigable in human time frames
- makes intergalactic travel plausible
- would relax the somewhat artificial and tortured Warp 10 limit, however, this can be restored in a more mathematically rigorous way (see below)
While the proposed speeds are significantly greater than the standard scales, at least for higher Warp values, they fit more comfortably with the vast distances found in our Universe. For example, using the old scale (TNG say), to travel from Earth to the centre of our galaxy, about 25,000 light years, it would take over 16 years at Warp 9 and over 3 years at Warp 9.99.
Going to another (close) galaxy is simply implausible, taking 300 or more years at Warp 9.99. Given there are hundreds of billions of galaxies out there, it seems a bit limiting to restrict exploration to such a tiny fraction of the known universe.
By contrast, Table 2 below shows a distance and travel time chart for the proposed new scale.
| Warp | Local Planets | Nearby stars | Visible stars | Federation | Centre of galaxy | Cross galaxy | Nearby galaxies |
|---|---|---|---|---|---|---|---|
| (0.0005 ly) | (50 ly) | (500 ly) | (5,000 ly) | (25,000 ly) | (100,000 ly) | (5 Million ly) | |
| 1 | 4.4 hr | 50.0 yr | 500 yr | 5,000 yr | 25,000 yr | 100,000 yr | 5,000,000 yr |
| 2 | 26.3 min | 5.0 yr | 50.0 yr | 500 yr | 2,500 yr | 10,000 yr | 500,000 yr |
| 3 | 2.6 min | 6.0 mth | 5.0 yr | 50.0 yr | 250 yr | 1,000 yr | 50,000 yr |
| 4 | 15.8 sec | 2.6 wk | 6.0 mth | 5.0 yr | 25.0 yr | 100 yr | 5,000 yr |
| 5 | 1.6 sec | 1.8 day | 2.6 wk | 6.0 mth | 2.5 yr | 10.0 yr | 500 yr |
| 6 | 0.16 sec | 4.4 hr | 1.8 day | 2.6 wk | 3.0 mth | 1.0 yr | 50.0 yr |
| 7 | 16 msec | 26.3 min | 4.4 hr | 1.8 day | 1.3 wk | 1.2 mth | 5.0 yr |
| 8 | 1.6 msec | 2.6 min | 26.3 min | 4.4 hr | 21.9 hr | 3.6 day | 6.0 mth |
| 9 | 0.16 msec | 15.8 sec | 2.6 min | 26.3 min | 2.2 hr | 8.8 hr | 2.6 wk |
| 10 | 0.016 msec | 1.6 sec | 15.8 sec | 2.6 min | 13.1 min | 52.6 min | 1.8 day |
Table 2 Distance and Travel Times for the Proposed New Warp Speeds
Remembering that a scale is just that, a scale. It doesn’t mean that starships can reach these speeds or sustain them for extended periods. Indeed, standard cruising speed may be significantly less than full speed because of engine stress and energy efficiency.
The next section builds on these ideas by placing limits on Warp speed based on various criteria.
Warp Speed Constraints
To provide limits on the speed of Warp capable ships, and to stay faithful to the idea of a universal maximum Warp speed, for example Warp 10, we can introduce some constraints. One approach is a blend of relativistic and newtonian mechanics.
First, let’s assume that:
- The force required to move a ship though the Warp field is proportional to the square of its Warp speed, and,
- There is an universal maximum Warp speed (
μ) similar to the speed of light in standard physics
Based on these two assumptions, the force (F) required to “push” through the Warp field can be written mathematically as:
| F = aw2 / √(μ2-w2) | …(2) |
| where: | |
| F = force | |
| a = constant | |
| w = warp speed | |
| μ = universal maximum warp speed |
The numerator is the Warp squared factor from the first assumption above. The denominator is analogous to the Lorentz factor of special relativity which is usually written 1/√(c2-v2). It means the required force increases as the speed approaches the universal maximum Warp speed and becoming infinite at that maximum Warp speed. This meets the second assumption above and provides a robust mathematical underpinning of the limit.
Using the force definition from Equation 2 and the velocity from Equation 1, we can calculate the Power (P) required by a Warp engine to maintain a constant velocity using the standard relation:
- Power (
P) = Force (F) by velocity (v)
This gives:
| P = Fv = acw210w-1 / √(μ2-w2) | …(3) |
| where: | |
| P = power | |
| F = force | |
| v = velocity (speed) | |
| a = constant | |
| c = speed of light | |
| w = warp speed | |
| μ = universal maximum warp speed |
From Equation 3 the energy needed to travel a particular distance can be derived using the standard definitions:
- distance (
d) = velocity (v) by time (t) - Energy (
E) = Power (P) by time (t)
This leads to the following energy equation:
| d = vt | |
| E = Pt = (Fv)t = F(vt) = Fd = adw2 / √(μ2-w2) | …(4) |
| where: | |
| E = energy | |
| P = power | |
| F = force | |
| v = velocity (speed) | |
| t = time | |
| d = distance | |
| a = constant | |
| w = warp speed | |
| μ = universal maximum warp speed |
In addition to power and energy calculations, we can derive an equation for efficient defined as the distance travelled per unit of energy consumed (similar to fuel efficiency for motor vehicles). The efficiency (eff) equation follows directly from Equation 4:
| eff = d/E = √(μ2-w2) / aw2 | …(5) |
Setting a Universal Maximum Warp (μ)
We can choose any Warp speed as the universal maximum, but let’s stay with convention and make μ=10.
The constant a in the above equations depends on the “units” we use to measure the various quantities. For simplicity, let’s make P=1 when w=1. We can put these values into the Power equation (3) above and solve for a as follows:
| 1 = ac12101-1 / √(102-12) | |
| 1 = ac / √(102-12) | |
| ac = √(102-12) = √99 | |
| a = √99 / c |
Then substituting a back into Equations 3 gives:
| Pμ=10 = √99w210w-1 / √(100-w2) | …(6) |
Using the same approach for the Energy and Efficiency equations, namely:
- Energy = 1 per unit distance (say 1 lightyear) when w = 1
- Efficiency = 1 (100%) when w = 1
We get:
| Eμ=10 = √99w2 / √(100-w2) per unit distance | …(7) |
| effμ=10 = √(100-w2) / √99w2 | …(8) |
Table 3 below shows comparative results for Power, Energy and Efficiency for various Warp speeds based on Equations 6, 7 and 8. It also includes the time it would take to travel one light year at the given Warp speed as a reference.
| Warp | Power | Energy per ly | Efficiency (%) | Time to travel 1 ly |
|---|---|---|---|---|
| 1 | 1 | 1.0 | 100.00 | 1.0 yr |
| 2 | 41 | 4.1 | 24.62 | 1.2 mth |
| 3 | 939 | 9.4 | 10.65 | 3.6 day |
| 4 | 17,370 | 17.4 | 5.76 | 8.8 hr |
| 5 | 287,228 | 28.7 | 3.48 | 52.6 min |
| 6 | 4,477,443 | 44.8 | 2.23 | 5.3 min |
| 7 | 68,269,794 | 68.3 | 1.46 | 31.5 sec |
| 8 | 1,061,319,933 | 106.1 | 0.94 | 3.2 sec |
| 9 | 18,489,527,619 | 184.9 | 0.54 | 0.32 sec |
Table 3 Comparative Power, Energy and Efficiency for Various Warp Speeds (μ=10)
These results show that:
- Power requirements rapidly (exponentially) increase as Warp speeds increase
- Energy (fuel) requirements modestly increase as Warp speeds increase
- Efficiency, which is essentially the inverse of energy consumption, reduces in line with energy increases
The massive power requirements speak to the difficulty of achieving higher Warp speeds. It confirms the understanding that significant technical advances are required to go just one step up the Warp speed ladder. It also supports the idea that even a fractional increase in Warp speed comes at a significant cost in terms of additional power requirements.
While the power demands are very high at higher Warp speeds, the energy consumption (i.e. fuel use) remains relatively modest (although increasing). This means the main technical challenge in Warp drive design is to convert the available energy at a high enough rate to provide the power demands needed to achieve a given Warp speed.
The energy efficiency figures can be interpreted, in starship terms, as light years per unit of fuel, for example antimatter. So you get, say, 100 light years per kilogram of antimatter at Warp 1, but only 24.6 at Warp 2, and this drops down to just over half a light year (0.54) at Warp 9, of course you cover that half light year in a fraction of a second!
Despite the rather hypothetical situation, the overall results are consistent with what we might expect from Starship pseudo physics or, perhaps, Warp drive mechanics.
Engine Power Levels
We can further expand on these results to show how the engine power output affects Warp speeds. Lets assume full speed – the rated top speed – is achieved when the engine is producing 100% of its rated power output. Table 4 below shows the corresponding Warp speeds associated with various percentages of this power output for different rated Warp speeds (leftmost column).
| Rated Warp | 20% | 40% | 60% | 80% | 100% | 120% | 140% | 160% | 180% | 200% |
|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 1.5 | 1.7 | 1.8 | 1.9 | 2.0 | 2.1 | 2.1 | 2.1 | 2.2 | 2.2 |
| 3 | 2.5 | 2.7 | 2.8 | 2.9 | 3.0 | 3.1 | 3.1 | 3.2 | 3.2 | 3.2 |
| 4 | 3.4 | 3.7 | 3.8 | 3.9 | 4.0 | 4.1 | 4.1 | 4.2 | 4.2 | 4.2 |
| 5 | 4.4 | 4.7 | 4.8 | 4.9 | 5.0 | 5.1 | 5.1 | 5.2 | 5.2 | 5.3 |
| 6 | 5.4 | 5.7 | 5.8 | 5.9 | 6.0 | 6.1 | 6.1 | 6.2 | 6.2 | 6.3 |
| 7 | 6.4 | 6.7 | 6.8 | 6.9 | 7.0 | 7.1 | 7.1 | 7.2 | 7.2 | 7.3 |
| 8 | 7.4 | 7.7 | 7.8 | 7.9 | 8.0 | 8.1 | 8.1 | 8.2 | 8.2 | 8.2 |
| 9 | 8.4 | 8.7 | 8.8 | 8.9 | 9.0 | 9.1 | 9.1 | 9.2 | 9.2 | 9.2 |
Table 4 Warp Speeds for Various Engine Power Percentages (μ=10)
Table 4 shows that running on lower power settings doesn’t significantly reduce the Warp speed compared with its rated full speed (100% power). And conversely, running the engine above its rated power (i.e. overloading it) produces only marginal gains in Warp speed.
Based on this observation, let’s assume Starfleet defines the following Warp speed/power designations:
| Designation | Percentage of Rated Power | Description |
|---|---|---|
| Reserve Warp | 10% | Energy conservation or damaged engine |
| Standard Warp | 33% | Standard cruising speed, default if no speed specified |
| Express Warp | 67% | Expedited speed |
| Full Warp | 100% | Rated full speed |
| Flank1 Warp | 133% | Flank speed (medium overload) |
| Maximum Warp | 167% | Maximum speed (maximum overload), emergency situations |
Table 5 Starfleet Designations with Associated Rated Power Percentages
- Flank is a US nautical term used to denote speeds in excess of “full” speed.
Using these Starfleet designations, the corresponding Warp speeds, and the time to travel one lightyear (ly) at those speeds, for different Warp rated ships are shown in Table 6 below.
| Rated Warp | Designation | Reserve | Standard | Express | Full | Flank | Maximum |
|---|---|---|---|---|---|---|---|
| Power % | 10% | 33% | 67% | 100% | 133% | 167% | |
| 2 | Warp | 1.3 | 1.7 | 1.9 | 2.0 | 2.1 | 2.2 |
| Time/ly | 5.4 mth | 2.5 mth | 1.6 mth | 1.2 mth | 4.3 wk | 3.6 wk | |
| 3 | Warp | 2.3 | 2.6 | 2.9 | 3.0 | 3.1 | 3.2 |
| Time/ly | 2.9 wk | 1.2 wk | 5.0 day | 3.7 day | 2.9 day | 2.5 day | |
| 4 | Warp | 3.2 | 3.6 | 3.9 | 4.0 | 4.1 | 4.2 |
| Time/ly | 2.3 day | 21.1 hr | 12.1 hr | 8.8 hr | 6.9 hr | 5.8 hr | |
| 5 | Warp | 4.2 | 4.6 | 4.9 | 5.0 | 5.1 | 5.2 |
| Time/ly | 5.8 hr | 2.2 hr | 1.2 hr | 52.6 min | 41.4 min | 34.3 min | |
| 6 | Warp | 5.2 | 5.6 | 5.9 | 6.0 | 6.1 | 6.2 |
| Time/ly | 36.3 min | 13.3 min | 7.4 min | 5.3 min | 4.1 min | 3.4 min | |
| 7 | Warp | 6.2 | 6.6 | 6.9 | 7.0 | 7.1 | 7.2 |
| Time/ly | 3.7 min | 1.3 min | 44.4 sec | 31.5 sec | 24.7 sec | 20.5 sec | |
| 8 | Warp | 7.2 | 7.6 | 7.9 | 8.0 | 8.1 | 8.2 |
| Time/ly | 21.7 sec | 7.9 sec | 4.4 sec | 3.2 sec | 2.5 sec | 2.1 sec | |
| 9 | Warp | 8.2 | 8.6 | 8.9 | 9.0 | 9.1 | 9.2 |
| Time/ly | 2.0 sec | 0.75 sec | 0.43 sec | 0.32 sec | 0.25 sec | 0.21 sec |
Table 6 Actual Warp Speed and Time to Travel 1 ly for Starfleet Designated Speeds (μ=10)
This means a starship that is rated as Warp 5, for instance, has a standard cruising speed of Warp 4.6 and maximum speed of Warp 5.2. However, there are two main reasons why ships cannot simply run at their maximum Warp speed:
- Energy cost (fuel)
- Engine endurance
We have already looked at energy consumption and seen that energy efficiency does reduce significantly at higher Warp speeds, but an even stronger reason to reduce Warp speed is engine endurance. This is explored in more detail in the next section.
Engine Endurance
Engine endurance is the length of time the engine can operate at a particular power output level before it requires maintenance. Typically Warp engines must be taken offline for a period to allow the maintenance to be performed.
Without maintenance, the risk of a major engine failure or even a catastrophic engine breach significantly increases.
I’m givin’ it all she’s got, Captain! If I push it any farther, the whole thing’ll blow!
– Montgomery ‘Scotty’ Scott
The higher the output power, and hence Warp speed, the shorter the operating time. Conversely, the lower the power output the longer the engine can run before requiring maintenance.
The engine endurance is a function of the ratio of actual engine power compared to the rated (100%) engine power (ρ = P / Pr). This ratio is one (1) when the actual power equals the rated power. Less than one when the actual power is lower than the rated power, such as cruising, and greater than one in overload situations.
There are various ways to formulate an endurance equation but let’s assume that Starfleet Warp drives have an endurance (ε) characterised by an inverted sigmoid function.
As a refresher, the following graphs show a “standard” sigmoid function and some progressive variations to transform it into something more suitable for use as an endurance function.
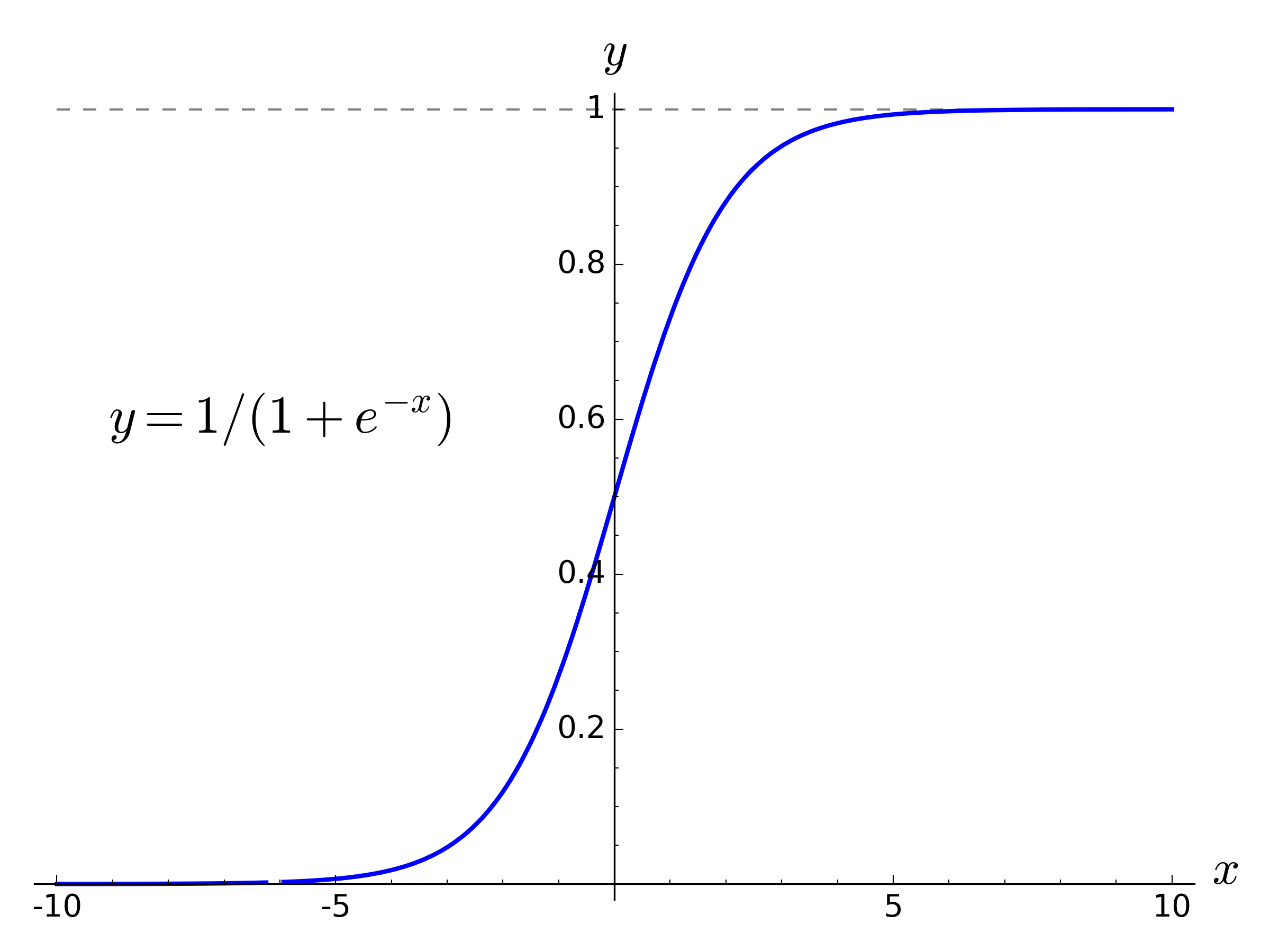
These versions use the natural logarithm base (e), rather than 10, say, but as shown below the actual base value is irrelevant.
For those not interested in the mathematical details, please skip to the Summary Table at the end of the post where all the results are summarised in one combined table.
The general form of the endurance equation is:
| ε(ρ) = a√(μ2-wr2) / (1 + bec(ρ-0.5)) | …(9) |
| where: | |
| ε = engine endurance | |
| a, b and c = constants (‘c’ is not the speed of light here) | |
| μ = universal maximum warp speed | |
| wr = rated Warp speed of the ship/engine | |
| ρ = p / pr | |
| p = actual engine power | |
| pr = rated engine full (100%) power |
The Lorentzian term √(μ2-wr2) in the numerator is designed to limit the endurance as the rated Warp speed (wr) increases. This changes the endurance characteristics for different rated Warp speeds. It makes the endurance approach zero as the rated Warp speed approaches the universal maximum Warp limit (μ).
By setting the exponent to ρ-0.5, the endurance curve is symmetric about ρ=0.5.
The constant a, in conjunction with √(μ2-wr2), forms the upper limit (asymptote) of the sigmoid function and becomes a scaling factor that depends on the units used to measure endurance.
The constants b and c can be determined by making the following assumptions:
- Endurance at half power (ρ=0.5) is half the maximum endurance (i.e. ε(0.5) = a√(μ2-wr2)/2). This also makes the maths below simpler.
- A Starfleet regulation states that for a Starship to be rated at a particular Warp speed (wr), its engine must be able to sustain that Warp speed for a minimum period of time (εr).
For brevity, we will assign k = √(μ2-wr2) in the follows calculations. Note k is a constant for each rated Warp speed.
Substituting Assumption 1, ε(0.5)=a√(μ2-wr2)/2=ak/2, into Equation 9 allows the value of b to be determined as follows:
| ε(0.5) = ak/(1 + bec(0.5-0.5)) = ak/(1 + be0) = ak/(1 + b) = ak/2 | |
| => (1 + b) = 2 | |
| => b = 1 |
Putting this value of b back into Equation 9 gives:
| ε(ρ) = ak / (1 + ec(ρ-0.5)) | …(10) |
Substituting Assumption 2, ε(1) = εr, into Equation 10 allows the value of c to be determined as follows:
| ε(1) = ak/(1 + ec(1-0.5)) = ak/(1 + ec/2) = εr | |
| => εr(1 + ec/2) = ak | |
| => εr + εrec/2 = ak | |
| => εrec/2 = ak – εr | |
| => ec/2 = (ak – εr)/εr = ak/εr – 1 | |
| => c/2 = log(ak/εr – 1) | |
| => c = 2log(ak/εr – 1) |
Putting this value of c back into Equation 10 above gives:
| ε(ρ) | = ak / (1 + e2log(ak/εr – 1)(ρ – 1/2)) |
| = ak / (1 + e(2ρ – 1)log(ak/εr – 1)) |
Which, remembering, enlog(x) = xn, simplifies to:
| ε(ρ) = ak / (1 + (ak/εr – 1)(2ρ-1)) | …(11) |
| where: | |
| ε = engine endurance | |
| a = constant unit scaling factor | |
| k = √(μ2-wr2) | |
| wr = rated Warp speed of the ship | |
| εr = endurance at full power (ρ=1) | |
| ρ = p / pr | |
| p = actual engine power | |
| pr = rated engine full (100%) power |
Finally, let’s provide some concrete values for μ, a and εr so we can calculate some actual results. We will stick with μ=10 for the universal Warp limit. Let’s use a (Earth) day as the endurance unit. Setting εr to 0.5 days (i.e. 12 hours) as the minimum period the engine must sustain the rated Warp speed.
Noting that k will range between 0 and 10 (mostly nearer 10), setting a to 10 will give a maximum endurance of 100 days. This seems about right. Substituting μ=10, a=10 and εr=0.5 into Equation 11 gives:
| ε(ρ) = 10√(100-wr2) / (1 + (20√(100-wr2) – 1)(2ρ-1)) days | …(12) |
| where: | |
| wr = rated Warp speed of the ship | |
| ρ = p / pr |
A plot of endurance at different rated Warp speeds is shown below:
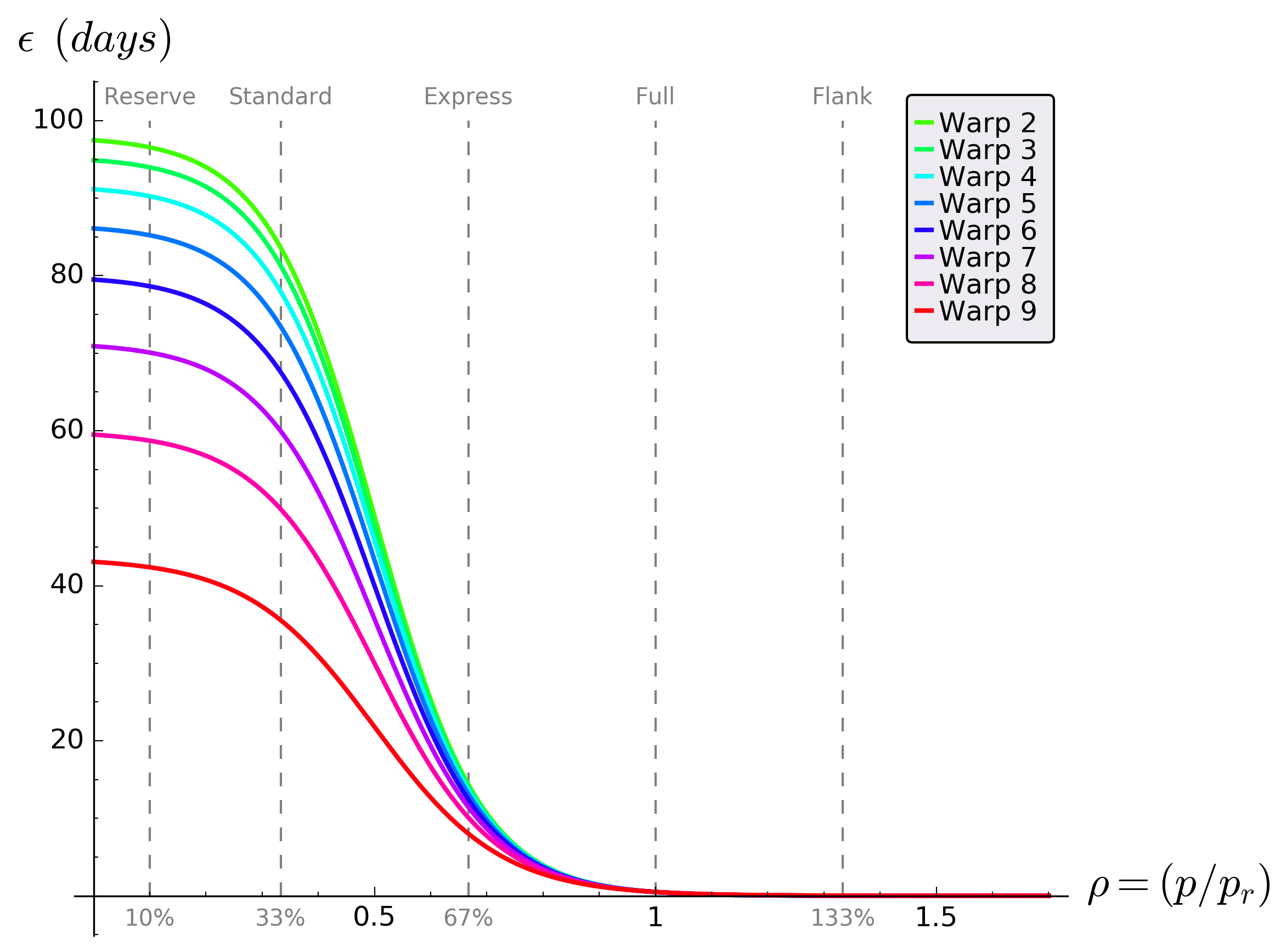
The following points can be drawn from this plot:
- Overall engine endurance declines progressively as the rated Warp speeds increase. Put simply, high performance engines need more frequent maintenance.
- All the individual lines meet at (ρ=1, ε=0.5), although it is hard to see in the plot.
- For any given rated Warp speed, the endurance declines significantly as the power ratio (
ρ) increases. In particular, running at standard (33%) power provides much better endurance than express (67%) or higher power ratios.
Table 7 below is a tabular version of the graph above. It shows engine endurance times for the designated power settings for different rated Warp speeds.
| Rated | Reserve | Standard | Express | Full | Flank | Maximum |
|---|---|---|---|---|---|---|
| Warp | (10%) | (33%) | (67%) | (100%) | (133%) | (167%) |
| 2 | 3.2 mth | 2.7 mth | 2.1 wk | 12.0 hr | 21.5 min | 38.4 sec |
| 3 | 3.1 mth | 2.7 mth | 2.0 wk | 12.0 hr | 21.9 min | 39.8 sec |
| 4 | 3.0 mth | 2.6 mth | 2.0 wk | 12.0 hr | 22.5 min | 42.0 sec |
| 5 | 2.8 mth | 2.4 mth | 1.9 wk | 12.0 hr | 23.4 min | 45.3 sec |
| 6 | 2.6 mth | 2.2 mth | 1.8 wk | 12.0 hr | 24.7 min | 50.4 sec |
| 7 | 2.3 mth | 2.0 mth | 1.6 wk | 12.0 hr | 26.6 min | 58.8 sec |
| 8 | 1.9 mth | 1.6 mth | 1.4 wk | 12.0 hr | 30.0 min | 1.2 min |
| 9 | 1.4 mth | 1.2 mth | 1.1 wk | 12.0 hr | 37.3 min | 1.9 min |
Table 7 Endurance Times for Different Rated Warp Speeds (μ=10)
Table 7 clearly shows that the endurance at full speed is the same (0.5 day = 12 hr) for each rated Warp speed in accordance with Assumption 2 above.
It also shows, which is not clear from the graph, that the engine endurance of overloaded power settings (i.e. flank and maximum) are dramatically less than full power, measured in only minutes or seconds. This plays into the idea that, in an emergency, it is possible to squeeze a bit more speed from the engine but only for comparatively short periods.
Summary
The various results above can be combined into a single summary table. Table 8 below shows the following information:
- Warp speed (at the designated power setting)
- Endurance (maximum time the engine can run before maintenance)
- Maximum distance before maintenance (Warp speed
xendurance time) - Time to travel 1 lightyear (at the specified Warp speed)
- Energy (fuel) to travel 1 lightyear in an arbitrary unit. In this case we have used kilograms of antimatter
for ships of different rated Warp speeds running at Starfleet designated power settings. The data is based on the equations developed earlier.
| Rated Warp | Designation | Reserve | Standard | Express | Full | Flank | Maximum |
|---|---|---|---|---|---|---|---|
| Power % | 10% | 33% | 67% | 100% | 133% | 167% | |
| 2 | Warp | 1.3 | 1.7 | 1.9 | 2.0 | 2.1 | 2.2 |
| Endurance | 3.2 mth | 2.7 mth | 2.1 wk | 12.0 hr | 21.5 min | 38.4 sec | |
| Distance | 0.59 ly | 1.1 ly | 0.30 ly | 0.01 ly | 0.00 ly | 0.00 ly | |
| Time/ly | 5.4 mth | 2.5 mth | 1.6 mth | 1.2 mth | 4.3 wk | 3.6 wk | |
| Fuel/ly | 0.2 kg | 0.3 kg | 0.4 kg | 0.4 kg | 0.4 kg | 0.5 kg | |
| 3 | Warp | 2.3 | 2.6 | 2.9 | 3.0 | 3.1 | 3.2 |
| Endurance | 3.1 mth | 2.7 mth | 2.0 wk | 12.0 hr | 21.9 min | 39.8 sec | |
| Distance | 4.6 ly | 9.7 ly | 2.8 ly | 0.14 ly | 0.01 ly | 0.00 ly | |
| Time/ly | 2.9 wk | 1.2 wk | 5.0 day | 3.7 day | 2.9 day | 2.5 day | |
| Fuel/ly | 0.5 kg | 0.7 kg | 0.9 kg | 0.9 kg | 1.0 kg | 1.1 kg | |
| 4 | Warp | 3.2 | 3.6 | 3.9 | 4.0 | 4.1 | 4.2 |
| Endurance | 3.0 mth | 2.6 mth | 2.0 wk | 12.0 hr | 22.5 min | 42.0 sec | |
| Distance | 40 ly | 88 ly | 27 ly | 1.4 ly | 0.05 ly | 0.00 ly | |
| Time/ly | 2.3 day | 21.1 hr | 12.1 hr | 8.8 hr | 6.9 hr | 5.8 hr | |
| Fuel/ly | 1.1 kg | 1.4 kg | 1.6 kg | 1.7 kg | 1.8 kg | 1.9 kg | |
| 5 | Warp | 4.2 | 4.6 | 4.9 | 5.0 | 5.1 | 5.2 |
| Endurance | 2.8 mth | 2.4 mth | 1.9 wk | 12.0 hr | 23.4 min | 45.3 sec | |
| Distance | 351 ly | 809 ly | 258 ly | 14 ly | 0.57 ly | 0.02 ly | |
| Time/ly | 5.8 hr | 2.2 hr | 1.2 hr | 52.6 min | 41.4 min | 34.3 min | |
| Fuel/ly | 1.9 kg | 2.4 kg | 2.7 kg | 2.9 kg | 3.0 kg | 3.1 kg | |
| 6 | Warp | 5.2 | 5.6 | 5.9 | 6.0 | 6.1 | 6.2 |
| Endurance | 2.6 mth | 2.2 mth | 1.8 wk | 12.0 hr | 24.7 min | 50.4 sec | |
| Distance | 3.1 kly | 7.3 kly | 2.4 kly | 137 ly | 6.0 ly | 0.25 ly | |
| Time/ly | 36.3 min | 13.3 min | 7.4 min | 5.3 min | 4.1 min | 3.4 min | |
| Fuel/ly | 3.1 kg | 3.8 kg | 4.2 kg | 4.5 kg | 4.7 kg | 4.8 kg | |
| 7 | Warp | 6.2 | 6.6 | 6.9 | 7.0 | 7.1 | 7.2 |
| Endurance | 2.3 mth | 2.0 mth | 1.6 wk | 12.0 hr | 26.6 min | 58.8 sec | |
| Distance | 27 kly | 65 kly | 22 kly | 1.4 kly | 65 ly | 2.9 ly | |
| Time/ly | 3.7 min | 1.3 min | 44.4 sec | 31.5 sec | 24.7 sec | 20.5 sec | |
| Fuel/ly | 4.8 kg | 5.8 kg | 6.4 kg | 6.8 kg | 7.1 kg | 7.4 kg | |
| 8 | Warp | 7.2 | 7.6 | 7.9 | 8.0 | 8.1 | 8.2 |
| Endurance | 1.9 mth | 1.6 mth | 1.4 wk | 12.0 hr | 30.0 min | 1.2 min | |
| Distance | 233 kly | 546 kly | 198 kly | 14 kly | 724 ly | 36 ly | |
| Time/ly | 21.7 sec | 7.9 sec | 4.4 sec | 3.2 sec | 2.5 sec | 2.1 sec | |
| Fuel/ly | 7.3 kg | 8.8 kg | 9.9 kg | 10.6 kg | 11.2 kg | 11.6 kg | |
| 9 | Warp | 8.2 | 8.6 | 8.9 | 9.0 | 9.1 | 9.2 |
| Endurance | 1.4 mth | 1.2 mth | 1.1 wk | 12.0 hr | 37.3 min | 1.9 min | |
| Distance | 1.8 Mly | 4.1 Mly | 1.6 Mly | 137 kly | 8.8 kly | 535 ly | |
| Time/ly | 2.0 sec | 0.75 sec | 0.43 sec | 0.32 sec | 0.25 sec | 0.21 sec | |
| Fuel/ly | 11.7 kg | 14.6 kg | 16.9 kg | 18.5 kg | 19.8 kg | 20.9 kg | |
| 9.5 | Warp | 8.8 | 9.2 | 9.4 | 9.5 | 9.6 | 9.6 |
| Endurance | 4.3 wk | 3.5 wk | 6.3 day | 12.0 hr | 46.9 min | 3.0 min | |
| Distance | 4.7 Mly | 9.9 Mly | 4.1 Mly | 433 kly | 34 kly | 2.5 kly | |
| Time/ly | 0.55 sec | 0.22 sec | 0.13 sec | 100 msec | 83 msec | 72 msec | |
| Fuel/ly | 15.8 kg | 20.9 kg | 25.3 kg | 28.8 kg | 31.9 kg | 34.8 kg |
Table 8 Rated Warp Summary Table
For example, consider a Warp 5 rated ship. At Standard Warp, it will travel at Warp 4.6 which it can sustain for 2.4 months, or just over 800 lightyears, before maintenance is required. At this speed, it will take 2.2 hours and use 2.4 kg of antimatter for each lightyear travelled.
In an emergency, it can travel at Warp 5.2 but only for 45 seconds.
It is also worth noting that Standard Warp provides the best total range for a given rated Warp (i.e. the maximum distance travelled before engine maintenance is required). This agrees with commonsense that there is a “sweet spot” that balances engine endurance and raw speed.
In this post we have:
- Proposed an alternative log base-10 Warp scale that more realistically reflects the vastness of the Universe and makes it more navigable in human time frames
- Developed Warp Power, Energy and Efficiency pseudo-equations by combining relativistic and classical mechanics which provides a robust mathematical underpinning of a universal Warp speed limit
- Introduced the notion of engine endurance to restrict the distance Warp capable ships can travel at various Warp speeds before engine maintenance is required
STAR TREK and related marks are trademarks of CBS Studios Inc.
This is quite interesting. I’ve played around with similar ideas, but not in the depth you have and going in a bit different direction. It’s not ready for publication in any form, though. Basically, however, I posit that there clearly are three “generations” of warp technology: 1) Cochrane’s warp drive, which is pretty much the standard V=c*W^3 (based on the warping of 3-dimensional space); 2) the TOS-period warp drive, introduced sometime between when the SS Columbia was lost at Talos IV and when Enterprise intercepted their message, incorporating a “fourth/time-dimension” both a) raising the exponent to 4 (hence V=c*W^4 — yes, I know — HERESY!) and b) introducing the possibility of time-travel in certain circumstances, as would be discovered at Psi 2000 and upon encountering the Black Star, which discovery would start further research into the hyperdimensional physics which would ultimately yield … 3) the “transwarp” drive which was *not* a “failure” (its “failure” was as a result of sabotage — Starfleet would never have built the Excelsior around an unproven technology which might have still needed kinks worked out, but whose basic ideas had to have been sound, and which — I suggest — became the “warp drive” of the TNG/etc. era [despite the claims by producers to the contrary]) and which, due to something about its nature, had a somewhat different exponentiation such that Warp 10 is indeed infinity. It’s a really ugly equation: V=c*W^4*(W^|(1-W)/(10-W)|)^(1/W). It is considerably faster than the traditional cubic-scale, but it’s in the same ballpark at the lower Warp Factors. At Warp 6 it approaches a full order of magnitude faster (1882 c vs. 216 c), but it does not start exponentiating out of sight until past Warp 8. Even then, it is consistent (my other big problem with Okuda’s TNG scale). Of course, Warp 8 is screaming at over 10,000 c. At Warp 9 (over 46,000 c), Scotty would be screaming! At Warp 10, no one can hear you scream! I do like your notion of rated engine endurances and efficiencies.
Good stuff.
Incidentally, to annotate my post:
1. The original V=C*W^3 equation was revealed at least as far back as the book The Making of Star Trek in 1968. I’m not sure if or when it was “canonized” by inclusion in an episode, but it is generally assumed.
2. The SS Columbia and Talos IV incident is from the original pilot episode, The Cage, which used such terms as “time warp factor” and has one of the characters exult that between Columbia’s loss 18 years earlier and Enterprise finding the survivors, the “time barrier” was “broken” and “You won’t believe how fast you can get home!”
3. The Psi 2000 incident is from the first-season episode, The Naked Time, at the end of which, due to an emergency “cold start” implosion of the warp engines in close orbit of a contracting planet exhibiting unstable gravitational forces, Enterprise finds itself moving backward through time by three days in about a minute subjective time. At one point Sulu says their velocity is “off the scale.”
4. The Black Star incident is from the first-season episode, Tomorrow is Yesterday, which opens with Enterprise in low Earth orbit a few days before 20 July 1969 after hitting a “black star” while headed in the general direction of Earth, and having to overload their warp engines to break away from the “black star’s” gravity well. By the end of the episode, Spock has figured out how to reverse the effect by heading into the Sun’s gravity well at high warp speed and then breaking away in a “slingshot,” which first sends it back her back in time then forward, back to their own 22nd century.
5. Excelsior’s transwarp “failure” as a result of Scotty’s sabotage is in the movie, Star Trek III: The Search for Spock.
6. Of course, TNG is Star Trek: The Next Generation, by which time so much has obviously changed that, despite the producers’ claims that it is the same warp drive as the original series but there had been a “recalibration” of the warp scale, things are obviously different. Most notably, although the original series occasionally depicted Warp Factors greater than 10, in the TNG era WF 10 was supposedly infinite speed.